IntMath Newsletter: Valentine’s, applets, maps
By Murray Bourne, 14 Feb 2019
14 Feb 2019
In this Newsletter:
0. Geometry of love
1. New on IntMath: 3 new interactives
2. Quirky measurements
3. Resources: Map projections
4. Math movies: Speed of light, puzzles
5. Math puzzle: Remainders
6. Final thought: Finding your soul mate
0. Geometry of love
Happy Valentine's, everyone! Here's a mathematical interpretation of the ubiquitous symbol of love.
Did you ever consider the geometry of the idealised heart shape used in countless Valentine's messages? It's just a square rotated 45°, connected to 2 semicircles.
1. New on IntMath
(a) Math Art in Code: Animated Moore Curve
 |
This is an animation of the Moore Curve, an example of a space filling curve which consists of continuous (one-dimensional) fractal lines that bend around in ever more intricate ways such that they eventually fill a (2-dimensional) square. |
This article from the journal Nature includes an interesting application of space-filling curves to energy storage devices:
Bioinspired fractal electrodes for solar energy storages
(b) Degree and roots of polynomial equations
The concepts behind polynomial equations are quite important in mathematics, but a lot of students get bogged down in the algebra, while not seeing the big picture
 |
I rewrote the opening section and have included an interactive graph, that helps explain the concepts. |
The rest of that chapter introduces the Remainder and Factor Theorems, which I've always felt were rather useless, since only a very limited subset of polynomial equations can be solved using those techniques (the degree has to be low, and the numbers have to be "nice" so factoring is easy. But what about the huge number of cases where the numbers don't lead to easy factoring by inspection (or trial and error)?
Of course we should sove such cases using computers!
(c) Euler's Identity exploration
Leonhard Euler was a brilliant Swiss mathematician who made many contributions to the fileds of physics, astronomy and engineering.
His famous identity, eiπ + 1 = 0, has captivated people (since it neatly involves seven fundamental mathematical symbols) and proved to be very useful for simplifying many mathematical processses.
 |
This page allows you to explore the concepts behind the identity eiπ + 1 = 0. Drag the point around the circle until you achieve the identity. |
THis page was a request from reader Owen.
2. Quirky measurements
 |
Each of these seemingly informal measurements actually have a scientific component to them. |
3. Resources - map projections
(a) Map Projections on the Web
Every 2D map of the world has some distortion, the inevitable result of trying to project a 3-D object onto a 2-D plane.
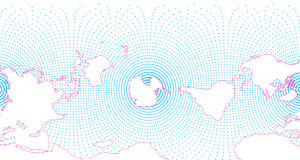
Some map types are better than others for preserving area, or shape, but none are prefect.
 |
GeoViz Studio has some great tools for exploring different projections. See especially the third (final) interactive example where you can choose from around 100 different projections. Go to: Map Projections on the Web |
GeoViz Studio also provides some great interfaces for creating your own map projections. See: Get Started Creating D3 Maps
These could be good starting places for students who want to learn how to code, via interesting challenges.
(b) Dymaxion Buckminster Fuller map
Inventor, architect, designer and futurist Buckminster Fuller proposed the Dymaxion projection in 1943.
Many of his designs were based on the geodesic sphere, an imaginary tesellation of a sphere into triangular pieces.
His Dymaxion Map is a projection based on the above sphere (actually basesd on an icosahedron).
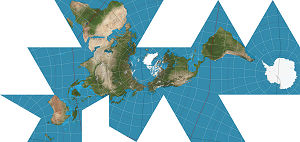
See also the results of a 2013 competition to re-invent Fuller's map:
7 Brilliant Reinventions of Buckminster Fuller's Dymaxion Map
4. Math Movies
(a) Speed of light
 |
These 3 simple NASA animations show how slow the speed of light really is, when we consider astronomical scales. This article is from Business Insider: |
(b) The joyful, perplexing world of puzzle hunts
 |
This video reminds us the human brain is wired to solve problems, and we experience a rush when we are successful, especially if it was challenging. |
One takeaway is we don't give students enough opportunity to create the problems in math class - they learn a lot by doing so!
5. Math puzzles
The puzzle in the last IntMath Newsletter asked "Who dies" in a scenario involving a rolling stone. Several conflicting answers were submitted. I added my own interpretation, which concludes D dies, but C may just have had a lucky day. Further comments are welcome!
New math puzzle: Remainders
Find the least two positive integers having the remainders 2, 3, 2 when divided by 3, 5, 7 respectively.
You can leave your response here.
6. Final thought - using math to find your soul mate?
Fewer people are meeting the love of their life in a "natural" way and are turning to apps to make connections for them. Algorithms continue to take over our lives and we all need to have a better understanding of the math behind such algorithms.
From Business Insider:
Here's how you can use math to find your soul mate — and why we're so resistant to that idea
Until next time, enjoy whatever you learn.
See the 17 Comments below.
