IntMath Newsletter - Math tools for school, world population

In this Newsletter:

1. Math tip (a) – Top math tools for school
2. Math tip (b) – Current world population; exponential growth
3. Latest IntMath Poll
4. From the Math Blog
5. Final thought – Keep it simple!

Each time I send out a mail there are a number of addresses that bounce back as "unknown address". Please add the IntMath Newsletter to your email "whitelist", so you don't miss an issue!

1. Math tip (a) – Top math tools for school

School is starting after a long summer break. Here are some tools and resources that you may find useful during the year.

a. Wolfram|Alpha
Cost: Free
Wolfram|Alpha is a "computational knowledge engine" that gives you a wealth of information about your math search. See my overview: Wolfram's Alpha

b. GraphSketch
Cost: Free
See my review at: GraphSketch.com free online math grapher

c. Algebra Problem Solver
Cost: You get the final answer free
You need to pay a subscription to see the explanation steps. Solves over 1 million problems a month for students.

d. Free math software downloads
Cost: As it says, "free"
This is a popular list.

e. DVD Math Videos
Cost: Moderate
Easy to understand explanations. Relaxed video lessons that explain a wide range of math topics. An 8-hour video course costs less than $30. If you order one of the bundled sets, it works out to $3.50 per hour.

f. Calculus Made Easy
Cost: Free
PDF version of an excellent explanation of calculus

g. Real-life math examples
Cost: Free
I get a lot of requests for real-life math examples, from students and teachers alike.

• Math Illuminated (See my overview of Math Illuminated.)
• Whos Counting John Allen Paulos examines the news through numbers (See my overview of Who's Counting)
• Twenty Global Problems and Twenty Years to Solve Them
• Earth killer - composite trigonometry CO2 graph

h. Free math books
Cost: As it says, "free"
Large list of free math books in PDF form

i. Interactive Mathematics
Cost: Free
Last but not least, hundreds of lessons in a wide range of topics from number and algebra through logarithms and trigonometry through to college-level calculus.

I hope you found something useful in that list!

2. Math tip (b) – Current world population, an example of exponential growth

On the home page of Interactive Mathematics I've got a "current world population" counter.

A reader was intrigued with this and asked if my counter was somehow linked to some global population database.

Well, no.

I have a script running in the background and it uses a best estimate for the population at a particular point in time (currently just under 6.8 billion) and applies the current estimated rate of growth (1.3% per year). So the population at time t (in years) is given by:

P(t) = 6,780,000,000 × (1.013)^t

This, of course, gives the net population (number of new babies minus the number of deaths).

This formula is an example of an exponential growth equation. See more at Exponential and Logarithmic Functions and especially the world population interactive.

To get the counter to tick over every time there is an increase in population by 1 person, the script divides the 1.3% per year increase by the number of milliseconds in a year (365.25 × 24 × 60 & times; 60 × 1000 =31557600000). It then triggers itself every 385.08 milliseconds (or just over 1/3 of a second), which is the time interval for the population to increase by 1.

Why do I have the counter so prominently displayed? Our planet isn't coping with the number of people it's got now. The evidence is hunger, collapsing fish stocks, inadequate clean water, pollution, global warming, overcrowding - the list goes on and on. We have to do something to reduce the present growth of around 1 billion people every decade.

This graph shows estimates for the world population from year 0 through to 2150 (based on UN Population Data). The good news is that the rate of increase has been dropping since 1960 (when it was over 2% per year) and it is expected to drop to around 0.5% per year by 2050, by which time there will be somewhere between 10 and 12 billion people trying to survive.

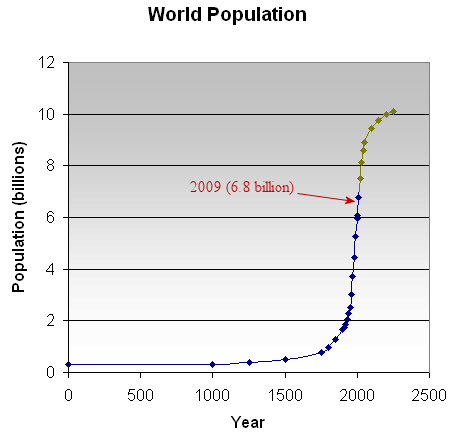
In the next chart, I have added the model P(t) = 6 × (1.013)^(t-2000), using the year 2000 as the base (in red). It is quite a good fit for the period from around 1800 to now.

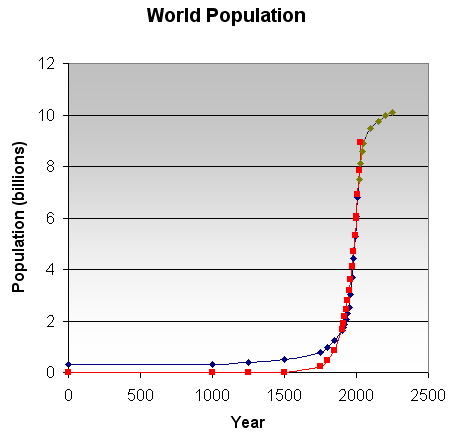
Next, we see what would happen if the current growth rate of 1.3% per year were to continue. By 2150 we would have over 40 billion people. What a nightmare.

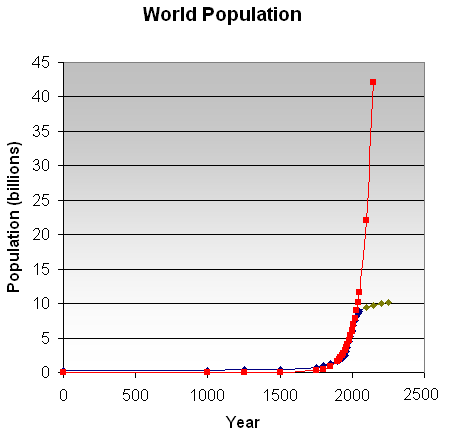
The study of populations is fascinating and an interesting career option. "Demographics" looks at particular aspects of populations (like aging) and is also a worthwhile field to pursue.

3. Latest IntMath Poll

The results for the latest IntMath Poll are in. Readers were asked how many books they intended to read over the summer holidays (aimed at those of you in the Northern Hemisphere, of course).

How is it relevant to math? Well, reading a lot means you understand better how the world works, and your vocabulary is better. So when you come across new concepts in math, you are less scared about the new words and can concentrate on the meaning of those new words.

The poll results:

32% One book per month

32% One book per week

22% No books

14% One book per day

The 22% who didn't read at all over the holidays have missed a great opportunity. Maybe next summer?

The new IntMath Poll asks readers about their hand phone use in class. This will be an interesting result! You can vote on any page in Interactive Mathematics.

4. From the math blog

a) A Mathematician's Lament - how math is being taught all wrong
Why is math boring for so many students?

b) When zombies attack - a mathematical model
This article uses math to predict what will happen if there is a zombie outbreak.

c) Math and the dating game - The Carol Syndrome
Probability explains that curse of the super-beautiful - it's hard to find a mate.

Final thought - Keep it simple

Have you ever realized that nearly all the math that you ever see in school involves just 4 things - addition, subtraction, multiplication and division? The day I figured that out was the day I stopped worrying about how difficult math seemed and I began to really enjoy it.

There is some truth in the following quote. Don't make life (and math) more complicated than it needs to be.

Any fool can make things bigger, more complex, and more violent. It takes a touch of genius — and a lot of courage — to move in the opposite direction.
~ Albert Einstein

Until next time, enjoy your new school year.

See the 14 Comments below.