Length of an Archimedean Spiral

By Murray Bourne, 21 Sep 2011

Reader Anantha recently wrote asking the following interesting question:

To find the total length of a flat spiral having outer end radius = 15.5 units, inner radius = 5 units & the increase in radius per turn = 0.81 unit, the total No. of turns in the spiral is 7.5.

This is an example of an Archimedean Spiral, otherwise known as an arithmetic spiral, where the arms get bigger by a constant amount for each turn.

We can see Archimedean Spirals in the spring mechanism of clocks and in vinyl records (used by the recording industry before CDs and MP3s); and in tightly coiled rope.

Archimedean Spiral
Watch mechanism [Image source: Hamilton Clock]

Archimedean Spiral
Coiled rope [Image source: Delderfield]

However, her question can't be solved

This was another example of an impossible-to-solve reader question. (Some of them are not possible to solve either because there is not enough information given, the algebra is unreadable, or some key vocabulary is not used correctly.)

In this case, it's not possible because it has a fundamental flaw.

If the inner radius is 5 units and the increase in radius per turn is 0.81 units, then 7.5 turns will give us an outer radius of:

5 + 0.81 × 7.5 = 11.075

So the outer radius cannot be 15.5 units if the increase per turn is 0.81.

Here's what the spiral with 0.81 radius increase per turn looks like:

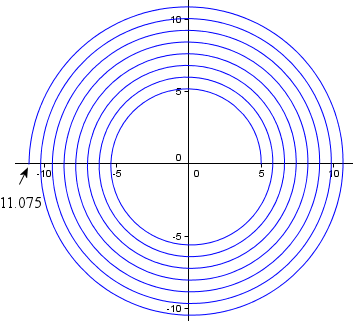
Archimedean spiral, 0.81 units between each arm;
outer radius is 11.075

On the other hand, if is it important to have the outer radius being 15.5 units, then the increase per turn would need to be:

(15.5 − 5)/7.5 = 10.5/7.5 = 1.4

Here's the spiral with inner radius 5, outer radius 15.5 after 7.5 turns:

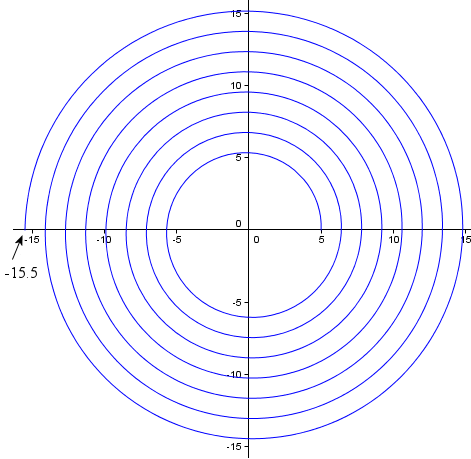
Archimedean spiral, inner radius 5, outer radius 15.5;
distance between each arm is 1.4 units

The increase per turn is 1.4 units.

Finding the Length of the Spiral

Before we can find the length of the spiral, we need to know its equation.

An Archimedean Spiral has general equation in polar coordinates:

r = a + bθ,

where

r is the distance from the origin,
a is the start point of the spiral and
b affects the distance between each arm.
(2πb is the distance between each arm.)

For both spirals given above, a= 5, since the curve starts at 5.

For the first spiral,

2πb = 0.81, giving us b = 0.12892

So the formula for the first spiral is

r = 5 + 0.12892θ

Using the same process,

2πb = 1.4, giving us b = 1.4/(2π) = 0.22282

So the formula for the second spiral is:

r = 5 + 0.22282θ

Length of the first spiral

We'll use the formula for the Arc Length of a Curve in Polar Coordinates to find the length.

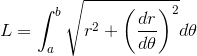
$\large{L=\int_{a}^{b}\sqrt{r^2+\left(\frac{dr}{d\theta}\right)^2}d\theta}$

The starting value for θ is a = 0 and after 7.5 turns, the end point is b = 7.5 × 2π = 15π = 47.12389.

The derivative under the square root is straightforward:

$\large{\frac{dr}{d\theta}=0.12892}$

Substituting everything into our formula gives us:

$\large{L=\int_{0}^{15\pi}\sqrt{(5+0.12892\theta)^2+(0.12892)^2}d\theta=378.8}$

You can see where that final answer comes from in Wolfram|Alpha. It may be possible to find the actual integral on paper (it involves hyperbolic functions), but why waste our lives doing so? We are interested in the length, not pages of algebra!

Length of the second spiral

I've solved the second one as an example in the section Arc Length of a Curve in Polar Coordinates.

So Anantha, I hope that helps to answer your question.

See the 60 Comments below.

Posted in Mathematics category - 21 Sep 2011 [Permalink]

Tags: Graphs, Technology

60 Comments on “Length of an Archimedean Spiral”

Sami A. Melki says:
21 Sep 2011 at 5:23 pm [Comment permalink]
Excellent .

These plane geometry equations are simple and beautiful .
James says:
22 Sep 2011 at 1:10 pm [Comment permalink]
The explanation is very clear.
brent says:
30 Apr 2012 at 8:54 am [Comment permalink]
Hi, thanks for this explanation.

I'm trying to work out the number of turns for a given length of spiral, (I'm an engineer for a garage door company, need to know turns to specify springs), I don't suppose you could give me some pointers getting N when I know L? I could use Solver in excel but I need to do it hundreds of times.

Brent.
Murray says:
1 May 2012 at 8:59 pm [Comment permalink]
@Brent: You can't solve it by any straightforward algebraic or calculus process - not that I can see, anyway.

Is your distance between each spiral always constant?

If so, I'm wondering if you can do it by getting a good approximation, then do a few actual calculations to zero in on the number of turns required.

For example, in the example in my post, the "average" radius of all the spirals is around 10 (1/2 way between 5 and 15). We know the length is 379, so the number of turns would be about 379 / (2 * pi * 10) = 6. This misses by quite a bit.

But a better "average" radius is 8 (obtained by working backwards from the known information), and this gives us the number of turns 379 / (2 * pi * 8 ) = 7.5.

Let's try 2 other lengths and see how we go, and I'm assuming our distance between spirals is constant at 1.4.

(1) Length 250 units. The average radius will be less - let's guess (for now) 7.5.

The number of turns will be 250 / (2 * pi * 7.5) = 5.3

Using this and the integration formula, gives 238, which is not bad for a reasonable guess. A small tweak or 2 would get you there.

(2) Going the other way, let's see what happens with length 500.

The average radius will be more, say 8.5.

Number of turns: 500 / (2 * pi * 8.5) = 9.4 turns

Applying the length formula, gives 520, which is once again pretty close. A few tweaks and we'll be very close.

Now, I don't know whether that would save you any work compared to what you are doing now. But maybe if you created a table with a bunch of "good" guesses and the resulting number of turns, and then used some kind of linear (or probably non-linear) interpolation, you would get close each time for a given length.

Does that help or am I describing what you're already doing?
brent says:
2 May 2012 at 8:26 am [Comment permalink]
Thanks for your reply Murray,

I'll describe what I ended up doing. I looked at the integral on the wolfram link in your blog post, saw it was a pretty regular shape - looks like a rectangle of width 2.pi.n and height average of top and bottom points. I put all this into an Excel table and used a data table to calculate length for n=1,2..10.

Then I graphed n-L and found that there's a quadratic trendline with and r^2=1 fit. So I used the linest function in excel to estimate the constants in the quadratic equations based on my table of n and L values, and used that to drive the rest of the excel table which calculates the required springs. (I used the quadratic equation formulae to find the roots of the quadratic. 5 years at uni learning maths, used it once now in 9 years of engineering...) I needed to have it in spreadsheet because I have to do this for every height and width combination of the door.

I couldn't have done any of that without your blog post. Thanks again. Brent.
Murray says:
2 May 2012 at 12:01 pm [Comment permalink]
Glad you got it to work, Brent.

And you're not the first engineer who has pointed out the lack of utility for much of the math learning at university. Of course, some engineers have got to know some of it, but maybe we need to re-think what is contained in the compulsory math components of engineering degrees.
brent says:
2 May 2012 at 2:16 pm [Comment permalink]
The thing about learning all the maths that you do in an engineering degree is not that you'll necessarily do all the things in your career, but that some of the subjects you'll do at uni require it. If you don't do the maths that lead up to it, then you're never going to be able to cope with thermodynamics or fluid mechanics. And you do need to be able to go through some of the maths in those subjects to truly say you understand the basic concepts.

The trouble is that there are simply so many fields that an engineer will go into, and you need to give an understanding of the fundamentals of just about everything: thermodynamics, fluid mechanics, structures, kinematics, control, electrical, materials etc etc etc. I might not have used the higher level maths much since leaving uni, but I've definitely used my understanding of all those subjects on a regular basis, and I gained my understanding by going through the maths.

What I would say to my 21 yr old self if I could: keep better track of your notes and text books, because it might be a decade before you need that information again but you will kick yourself if it's lost forever.
Neil says:
4 May 2012 at 7:50 am [Comment permalink]
Brent,

I hope this helps. It is a couple days late. If you know your overall length (OL), ID of the spiral, and OD of the spiral then the equation for the number of turns (N) is:
N = OL/(PI*(ID+OD)).

Also, on the original posting with the spacing of .81 units, the OD of the spiral should have been 11.075 units not 10.67 units. I think you solved for 7 turns, not 7.5 turns.
Murray says:
5 May 2012 at 12:54 pm [Comment permalink]
Thanks for your input, Neil.

Hmmm - seems a lot simpler that what Brent and I were talking about!

Thanks for spotting the error - I have amended the post.
brent says:
7 May 2012 at 7:19 am [Comment permalink]
Neil,

Thanks a lot for the information.

As it turns out for a garage door I don't know the OD - it's going to change depending on N.
Jacob says:
8 Nov 2012 at 6:08 pm [Comment permalink]
This is a bit late, but new readers may still find it usefull.

Another way to make a quick approximation is to take the average 🙂 For the first spiral in the article, inner radius is 5, and outer radius is ~ 11. The average radius is then (5+11)/2 = 8. The circumference of a circle with radius 8 is 2*pi*8 = 50.27. 7.5 turns of that length is 8*50.27 = 377.25. This, IMHO at least, is reasonably close to the 'exact' 378.8.

No matter how you look at it, you need three of ID, OD, N and L to compute the fourth - you cannot 'just' deduce N from L if you dont know ID and OD. But knowing ID, OD and L, you can quickly make a good estimate based on circles with diameter (ID+OD)/2.

It is a little different if you have the increase per turn. Say you have a roll of tape and know ID, tape thickness t and tape length L. You can then use the following formula to compute the number of turns (or layers, if you will):

N = (t - ID + sqrt( (ID-t)^2+(4*t*L/pi) )/(2*t)

and from this you can also compute the outer diameter OD

OD = 2*N*t+ID

Note, that both of the above formulae are approximations based on concentric circles, rather than a true spiral. However, the end result is reasonably close. Also note that it is diameters, not radii, you need to plug in!
Murray says:
9 Nov 2012 at 11:36 am [Comment permalink]
@Jacob - Thanks! We get closer and closer to a good approximation with each new post.
Rafael says:
25 Jan 2013 at 9:40 pm [Comment permalink]
Hi thanks a lot for your highly explanatory blog-entry! I still tried to solve the integral and after petty substitution I ended up with an integral of the form sqrt(x^2+1) ... which can be solved relatively easily once you know the trick (see http://www.matheboard.de/archive/30730/thread.html for example) ... however, in the blogpost from "wolfram" the integral is given by a much more complex formula (http://mathworld.wolfram.com/ArchimedesSpiral.html ) ... now I was wondering if you have any idea where my error lies with the relatively simple method? best regards and please keep up the good and informative posts 🙂
Ray Brown says:
15 Apr 2013 at 1:58 am [Comment permalink]
Ok I'm trying to solve something for a fiction story and it's been years since I've done calculus. So maybe someone can solve this for me. If you wanted to travel to the galactic core from Earth you would want to travel down the spiral arm and not through the less dense regions if you needed to resupply. So Earth is about 7900Kpc from the galactic core. The start of the spiral arm at the end of the galactic bar is about 3000Kpc from the core. I do not know the distance between the arms. What I'm trying to figure out is how far you would travel down the spiral arm to get to the galactic bar. Any help would be greatly appreciated. Thanks.
Murray says:
16 Apr 2013 at 12:10 pm [Comment permalink]
Hi Ray

I think the dotted magenta distance is what you mean from your description. Before we go any further, is it correct?

[Image source: UniverseToday]
brent says:
16 Apr 2013 at 12:13 pm [Comment permalink]
I think for something with the precision like this you could use the pythagorean approximation just fine.
Chris Gavin says:
27 Apr 2013 at 11:13 pm [Comment permalink]
Hello,
I'm not a mathemetician, but I have an archimedian spiral question too.
I would like to create a plastic spiral for developing long lengths of cine film. If the film length is 15 metres (15000mm), and the inner radius of my spiral should be 20mm, and the outer radius maybe 150mm. Ideally I would like about 2mm spacing between the winds. How many turns would there be?

The spiral will always need to be 15metres long, but I might like to experiment with different radii, spacing and number of turns etc. is there a formula that helps me do the maths for this please?

With the answer, I should be able to draw the correct spiral in Adobe Illustrator, and from there, create the plans needed to have the spiral made out of plastic...

Many thanks if anyone can help.
Robert Livesey says:
4 Oct 2013 at 11:09 pm [Comment permalink]
If spiral equation is r = a + b*Theta, where a is start radius
Spiral length is L = (1/b)Integral(sqr(r^2 + b^2))dr

Solution:
L = .5*[r*sqr(r^2 + b^2) + b^2 * log(r + sqr(r^2 + b^2))]/b

In Excel, in the VB code pages add a module and enter following code:
Option Explicit
Const cPi As Double = 3.14159265358979

Public Function LSpiral(ByVal Theta As Double, Pitch As Double, Start As Double)
Dim b As Double
Dim r As Double

b = 0.5 * Pitch / cPi
r = Start + b * Theta
LSpiral = Integral(r, b) - Integral(Start, b)
End Function

Private Function Integral(x As Double, b As Double)
Dim Tmp As Double

Tmp = Sqr(x ^ 2 + b ^ 2)
Integral = 0.5 * (x * Tmp + b ^ 2 * Log(x + Tmp)) / b
End Function

Then in the spreadsheet have 3 cells for Theta, Pitch and Start - you could have a cell for Number of turns and get theta from Turns * 2Pi
You can play with values to get the length you want
Murray says:
6 Oct 2013 at 11:11 am [Comment permalink]
@Robert - thanks for the Excel-based approach.
R.A,Maxwell says:
10 Jan 2014 at 11:41 pm [Comment permalink]
hi
I am a co op student and i am trying to make a roll of scotch tape in NX 7.5. I am attempting to use Law Curve to create a spiral line for an extrude to follow. how does the math (r = a + b?) equation or formula flow to create this? the roll needs to be roughly 15 mm thick and the total radius is 32.2 mm.
Murray says:
13 Jan 2014 at 5:16 pm [Comment permalink]
?Hello R.A.

This sounds like an interesting problem. Just to clarify a few things:

(1) What does "NX 7.5" mean?

(2) Does that mean the inner radius is 17.2 mm (since the roll itself is 15 mm thick and the total radius is 32.2?)

(3) How thick is the scotch tape? (I'll assume 0.07 mm, which I found here: http://www.tedpella.com/tape_html/tape.htm)

With these assumptions, we have

a = 17.2 (the inner radius)

b = (0.07 * number of turns)/ (2 pi)

So all together, the formula for your spiral for an angle theta would be:

r = 17.2 + ( (0.07 * number of turns)/ (2 pi)) theta

Does that help to get you started?
Singh says:
23 Apr 2014 at 7:45 pm [Comment permalink]
Hello Mr. Murray,

R.A means nx7.5 software. My query is similar. I want to draw a arithmatic spiral with the help of law curve in nx7.5. Pl. help. Thanks.
Murray says:
24 Apr 2014 at 10:56 am [Comment permalink]
@Singh: Thanks for the heads up about NX7.5. It looks like great software.

You just need to use the same formula, r = a + b θ, as explained in the article. You can use t instead of θ.
Manufacturing Student says:
16 Jul 2014 at 5:45 am [Comment permalink]
Hi Murray,

I have found this page extremely helpful and your explanations are very crispy. I have a very simple problem, hope you can give your valuable input:
I have to turn a disc on lathe machine. The disc is rotating at 500 rpm. This disc has an Outer Diameter of 70 mm. The tool that will be used to turn this disc is advancing radially toward the disc with a feed rate of 0.1 mm /rev (this simply means the thickness of chip (or shaving) removed from disc would be 0.1 mm). I want to know that if after machining the dia of dic is reduced by 2 mm i.e. it has become 68 mm than what spiral length of material is removed from the disc. And secondly lets say that I want to remove the 2000 mm of spiral length of material from disc in my experiments and I know the starting diameter than is there any formula that I can calculate the dia of disc that would result after removing this much spiral length of material. Thanks
Murray says:
30 Jul 2014 at 6:36 pm [Comment permalink]
Hello

You just need to use the same formula given in the article.

For your spiral, the radius will be given by:

${\int_{a}^{b}}\sqrt{{{r}^{2}+{{\left({\frac{{{d}{r}}}{{{d}\theta}}}\right)}}^{2}}}{d}\theta$

$={\int_{0}^{31.4}}\sqrt{{{{\left({35}-{\frac{{{0.1}\theta}}{{{2}\pi}}}\right)}}^{2}+{{\left(-{\frac{{{0.1}\theta}}{{{2}\pi}}}\right)}}^{2}}}{d}\theta$

To make sure it makes sense, let's first do 5 spirals:

For 5 rounds:

5 × outer circumference = 5 × (2πr) = 5 × (2π × 35) = 1099.5574

It makes sense, as our distance is slightly less than if it was 5 times around the outer rim.

For the case given, 68 mm final diameter means 10 revolutions, or 20π. The spiral length is:

${\int_{0}^{{20}\pi}}\sqrt{{{{\left({35}-{\frac{{{0.1}\theta}}{{{2}\pi}}}\right)}}^{2}+{{\left({\frac{{{0.1}\theta}}{{{2}\pi}}}\right)}}^{2}}}{d}\theta={2168.0047}$

I don't believe there is a formula for the other way around. But trial and error is quick and easy when using a computer algebra system.

So the amount of disk shaved is

9.215 × 0.1 = 0.9215
Manufacturing Student says:
31 Jul 2014 at 12:57 am [Comment permalink]
Thanks Murray

It makes a lot of sense. I will use it in my experimental plan and will let you know.

Thanks a lot for your valuable time and effort

Regards
raul says:
25 Aug 2014 at 8:19 pm [Comment permalink]
hi Murray and people! maybe you can help me!
this is for an opensource hw project!
im trying to design a pcb heated bed for a 3d printer. my printer is a delta
This is basically a copper spiral path.
I want to define the overall Resistance and seize: OD (ID could be zero). Since the material is copper and given the standard pcb layer height of 35 um, this resistance just depends on the section width. Lets call it E.
This Width could be aproximated by taking the increase per turn, so
R = e*W*L = e*b*L

i'm trying to come up with some formula o procedure to be able to calculate
>spiral number of turns
given
> OD
> resistance

any ideas?
thanks so much!
R

maths with a purpose are such fun!
Hiep says:
26 Aug 2014 at 12:57 am [Comment permalink]
using calculus, the answer is quite easy:
let s be length of an circular arc. Then
s = rθ; where θ is angle the arc spans, r=arc radius

In differential form we can write.
ds = rdθ then
s=integration(rdθ) (from 0 to arc angle)

Note that if r is constant, arc angle is 2*pi, then the integration will yield circumference of a circle,2*pi*r

Back to the spiral case:
r = r0 +n*θ where n is spiral constant, r0 is inner radius, r is spiral radius at any angle.

substitute r into ds equation and do integration, we have

spiral length = r0*θ + n*(θ^2)/2

Note: θ is in radian so for example if we have 10 turns spiral, then θ = 20*pi.

to find a spiral length from one angle to another use:

r0*(θ1-θ2) + n*(θ2^2-θ2^2)/2
Leah says:
7 Jun 2015 at 2:32 am [Comment permalink]
is this a typo?
r0*(θ1-θ2) + n*(θ2^2-θ2^2)/2

(θ2^2-θ2^2) = 0
merry gil arroyo says:
11 Oct 2016 at 8:27 pm [Comment permalink]
How can i get the value of increase in radius?
Murray says:
12 Oct 2016 at 8:21 am [Comment permalink]
@Merry: Near the top of the article, it says "the distance between each arm is 2πb divided by the number of turns".

Do you know your a and b values in this formula?

r = a + bθ.
Clive Pottinger says:
7 Jul 2017 at 9:44 pm [Comment permalink]
Mr. Bourne

I was trying to solve a problem very similar to the 'galactic spiral' problem posed by Ray Brown. In my case I was thinking of an airplane travelling from a ground point A to ground point C climbing steadily until it reached it's highest altitude H at the midpoint B. The distance travelled from A to B would be an Archimedean spiral and this is the distance I wished to calculate.

However, it dawned on me that I had already calculated the great circle distances for A to B at ground level (gcg) and for A to B at the altitude H (gch). In other words I new how far a car would have to travel along the ground from A to B (gcg), and I knew how far a bird at altitude H would have to travel from A to B (gch). One can then think of the distance travelled by the airplane as the same as that of the car but with the horizontal scale smoothly changing from gcg to gch as it progresses. So the distance travelled would be the average: (gcg + gch) / 2.

So, then I thought of applying that to spirals.
It seems to me then that the length of a spiral segment covering θ degrees could be expressed as the length of the circular arc of θ degrees with a radius that is the average of the radii of the segment's end points.

Am I wrong? My math is not nearly good enough to be sure.
Murray says:
23 Oct 2017 at 10:36 am [Comment permalink]
@Clive: Sorry about the delay in responding. This may be too late, but for what it's worth, here are some images that may help.

Let's assume our aircraft is partially going around the Earth, which I've drawn with radius = 1. The altitude of the aircraft is 0.5 (totally unrealistic, I know, but exaggerated so we can see what's going on).

One possible way to think about it is that the aircraft climbs and descends at a constant rate (in the x-y sense, relative to the horizon at that point), something like this:

The total distance traveled is:

2 × 0.8 + 1.3 = 2.9

However, aircraft take off horizontally, so maybe we should use tangents the the Earth, giving us this:

The distance travelled in this interpretation is:

2 × 1.12 + 0.32 = 2.56

Another possibility is your spiral suggestion (the second spiral is a bit off in my diagram).

This gives us a total flight of (very approx):

2 × 1.2 + 0.27 = 2.6

A third possibility is to consider the ascent and descent portions to be insignificant for the entire flight (we consider it just goes straight up), and just add the vertical climb plus ground distance. In this case we get:

2 × 0.5 + 1.9 = 2.9

Now to your final suggestion - taking the average of the 2 circular arc distances:

The distance now is 2.37.

I'm not sure we can conclude anything so far, but just wanted to indicate some possibilities.

BTW, I did the above diagrams using GeoGebra. It's an invaluable tool if you want to play with such questions, without getting bogged down in algebra.
Leo's Friend says:
29 Mar 2018 at 9:36 pm [Comment permalink]
Two scenario questions from someine who, obviously, is mathematically ignorant. The first concerns spirals made up of a set total length of "coil" and set distance between turns. The second may represent an infinite-length "coil" of percentage-proportionate distances.

Firstly, does your formula work for spirals going down to [tetminating at "a central-core point] zero (if such is accurately possible)? If such For example, if the spiral has 10 full turns, each turn is separated at an equal distance of 1. Would the formula work to determine the diffetent lengths of each full turn?

Secondarily, what if the distance between each turn differs by a static percentage relative to the length? Example, if the length of the first full turn is 10, the distance between its start point and the start point of the next inner turn is 1 (10% of the length). Ten, the distance between the 2nd and 3rd points would be 10% of the length of the 2nd full turn. Likewise, the distance between the 3rd and 4th would be 10% of the 3rd's full trun length. Etc, etc. Is there a formula yo determine the lengths of each arm in the series? I assume a spiral such as this would never realy achieve zero, but continue reducing in turn length and distance at each full turn.
Murray says:
30 Mar 2018 at 5:24 pm [Comment permalink]
Yes, you can use the formula to find the length of single spirals.

I'm not sure about your second case - I somehow doubt there will be a smooth join for each new arm of the spiral.
francesca says:
2 Apr 2018 at 5:55 am [Comment permalink]
Hi there, I am trying to find the equation to a spiral I have but I havent been able to understand how to find parameter b.

My spiral has an outer radius of 14 and an inner radius of 2.05. The parameter a=1.7
I dont know if its necessary but the spiral has 10 turns.
Is there any way I can find parameter b with this info?
Murray says:
2 Apr 2018 at 9:55 am [Comment permalink]
@Francesca: Since your inner radius is 2.05, it means a = 2.05.

outer radius − inner radius = 14 − 2.05 = 11.95.

In the article under the formula, it says (after I made a correction):

"2πb is the distance between each arm."

This means:

2πb = 11.95/10 = 1.195

and solving this gives:

b = 1.195/(2π) = 0.1902

So the equation for your spiral will be:

r = a + bθ

r = 2.05 + 0.1902θ

In this image (made using GeoGebra) you can see it goes from 2.05 to 14 in 10 spirals.

Hope it helps.
Mark says:
25 May 2018 at 2:58 am [Comment permalink]
With a clock hairspring the inner diameter and outer diameter of the coils remains constant at it’s attachments but so does the length of this spiral. So as the inner part of the coil is wound or unwound as in a watch then pitch cannot remain the same.
Murray says:
8 Jun 2018 at 3:56 pm [Comment permalink]
@Mark: Yes, in the case of a watch spring I imagine it will only form a spiral when it is completely unwound. In the wound state, the outer ring of the spiral becomes more straight, and the inner rings become more circular.
A7 says:
12 Jun 2018 at 11:53 pm [Comment permalink]
So.. I cannot solve In _0,^15pi in my Powercalc(my android device) D:
Murray says:
14 Jun 2018 at 5:18 pm [Comment permalink]
@A7: Do you mean ${{\left[{\ln{{\left({0}\right)}}}\right]}}^{{15}\pi}$ ? Actually, you won't be able to solve that on any calculator because ${\ln{{\left({0}\right)}}}$ is not defined.
Alex says:
21 Jun 2018 at 1:54 am [Comment permalink]
Can you solve for the amount of rotations when the only given information is the inner radius, the length between each arm, and the total length?
Murray says:
21 Jun 2018 at 11:35 am [Comment permalink]
@Alex: Please see Neil's comment (#8). I believe his formula should help you.
Mark says:
10 Aug 2018 at 8:03 am [Comment permalink]
Hopefully, one will have an easier time by sticking with polar coordinates and not sneaking in rectangular / Cartesian ones. Starting in the same way with the equation of an Archimedean spiral, in this case one that does not start at the origin because ${a}\ne{0}$ :

${r}={a}+{b}\theta$

To find the length of this curve from $\theta={0}$ to $\theta={2}\pi{N}$ , where 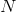is the number of turns of the spiral, leave the differential element 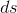of the curve as an infinitesimal circular arc instead of using a straight line segment, 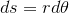${d}{s}={r}{d}\theta$ , then:

${L}={\int_{0}^{{2}\pi{N}}}{r}{d}\theta={\int_{0}^{{2}\pi{N}}}{\left({a}+{b}\theta\right)}{d}\theta={{\left[{a}\theta+\frac{{{\left({b}{\theta}^{2}\right)}}}{2}\right]}_{0}^{{2}\pi{N}}}$
Murray says:
10 Aug 2018 at 9:06 am [Comment permalink]
@MArk: Thanks for the alternate approach! I'm a big fan of simpler is better...
Abugheneda says:
16 Dec 2018 at 5:35 pm [Comment permalink]
Dear Engineers:
this post helped a lot and I am glad that one can find such a valuable information.
I have similar problem with different inputs.I have a disc rotating with certain r.p.m and a pin advancing with constant speed (mm/s) I know the staring Diameter and the stopping.
could I calculate the length of the track? and what effect the diameter of the pin have on the resulting length?
Murray says:
17 Dec 2018 at 3:10 pm [Comment permalink]
@Abugheneda: Yes, your situation can be calculated using the formulas given in the post. For the last part, I don't think the pin's diameter will make any difference to the length, unless the pin is very thick so that it affects how far apart each spiral is.
Abugheneda says:
21 Dec 2018 at 6:11 am [Comment permalink]
@Murray thanks a lot I will give you feed back with the resulting machine working.....
Mark says:
1 Jan 2019 at 4:57 am [Comment permalink]
@Murray: You are welcome. One thing i should point out, that got me stuck a bit, is if you are integrating say vectors at each point of this spiral curve (e.g, forces) then leaving them in purely polar form will not work. These vectors have to be projected into their components since integration is a simple addition type operation, while adding vectors uses a parallelogram law except for vector components that lie on the same axes. To bad because it makes for some very,very long equations when applying Castigliano's method to an Archimedean spiral spring.
Abugheneda says:
8 Jan 2019 at 6:11 pm [Comment permalink]
@Murray thanks for your help just one question
since my spiral goes from outside towards the center
will my equation be 'r=a-b*θ'?
Murray says:
9 Jan 2019 at 8:53 am [Comment permalink]
I believe it would be correct. The distance will be the same whether you are thinking about it stating from the outside moving in, or inside moving out.
Chris Zuniga says:
16 Jan 2019 at 11:18 am [Comment permalink]
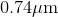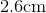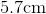@Murray Hello nice article. Mind lending me a help please?. I'm having some problems at trying to use your formula for calculating the length of a spiral in a CD. In my book the spacing between each arm is given as $0.74 \mu\textrm{m}$ and the width of the reading sector spans between $2.6\textrm{cm}$ to $5.7\textrm{cm}$ .

To calculate the number of turns what I did was to divide the width of this sector by the spacing:

$\frac{\left(5.7-2.6\right)\times 10^{-2}}{0.74\times 10^{-6}}= 41891.89189\,\textrm{turns}$

But for purposes of better precision I left it as indicated.

By going to your formula since:

$r=a+b\phi$

In my case:

$2\pi b=0.74\times10^{-6}$

$b= \frac{0.74\times10^{-6}}{2\pi} = \frac{37\times 10^{-8}}{\pi}$

and:

$a=2.6\times 10^{-2}=0.026$ , which is the distance from the origin.

In order to get the ending point of the integral you mentioned to

$2\pi\times\textrm{number of turns}$ $=2\pi\times \frac{\left(5.7-2.6\right)\times 10^{-2}}{0.74\times 10^{-6}}$ $=\frac{31\times 10^{5}\pi}{37}$

So with this upper boundary is established.

Appending the obtained constants my equations becomes into:

$r= 0.026 + \frac{37\times 10^{-8}}{\pi}\phi$

Therefore the required differential would be:

$\frac{\mathrm{d} }{\mathrm{d} \phi}\left(0.026+\frac{37 \times 10^{-8}}{\pi}\phi\right ) = \frac{37 \times 10^{-8}}{\pi}$

all that was left to do was to plug in the integral:

$\int^{\frac{31\times 10^{5}\pi}{37}}_{0}\sqrt{\left(0.026+\frac{37\times 10^{-8}}{\pi}\phi\right)^{2}+\left(\frac{37\times 10^{-8}}{\pi} \right )^{2}}d\phi$

Now if I followed your steps correctly this solved by Wolfram Alpha renders:

$\int^{\frac{31\times 10^{5}\pi}{37}}_{0}\sqrt{\left(0.026+\frac{37\times 10^{-8}}{\pi}\phi\right)^{2}+\left(\frac{37\times 10^{-8}}{\pi} \right )^{2}}d\phi$

Note: I couldn't make it to work with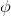 $\phi$ so instead I used 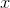as a variable. This part is odd, well I'm not very familiar with the use of Wolfram Alpha.

Here is the link from Wolfram Alpha

this other version features a more "expanded" format (not simplified fractions in the formula

So the result is a value very close to what can be obtained from a formula given above.

$L=\frac{\pi\times\left(R^2_{outher}-R^2_{inner}\right)}{separation}$

which in my case would be:

$L=\frac{\pi\times\left(0.057^2-0.026^2\right)}{0.74\times 10^{-6}}= 10923.4$

Both answers seem to check with what my book says the answer.

However I don't understand in your example

Why the formula for the second spiral is given as:

$r= 5 + 0.22282\theta$

Where the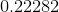 comes from?

If I were to calculate this for my problem what would be the value of the second spiral?

Why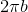 $2\pi b$ is the distance between each arm and not just .

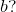When I followed your steps I felt mostly as reading a cookbook but I was left with the impression of keep investigating into this. At the end of your article you mentioned something about that the actual integral on paper would require hyperbolic functions. Does it mean that it cannot be solved using the ordinary integral methods found in most textbooks?. I'd like to know how could that integral be solved without much fuss as this left me intrigued.

I've attempted to reproduce the integral by replacing with letters A and B as constants but Wolfram Alpha rendered the results with log and none involving hyperbolic functions. Could it be that what I did was wrong?

I'll appreciate so much that you could help me clearing out these doubts.
Murray says:
18 Jan 2019 at 12:27 pm [Comment permalink]
@Chris: I'm impressed you entered all your math using LaTeX!

To answer your questions:

(1) Where the 0.22282 comes from?

I amended the post to more clearly indicate where that value came from.

(2) If I were to calculate this for my problem what would be the value of the second spiral?

I'm not sure what you mean here. I discussed 2 spirals in the post, but you only appear to be talking about one?

(3) Why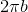 ${2}\pi{b}$ is the distance between each arm and not just ?

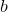I have seen it written both ways. I happen to be using the first.

(4) Does it mean that it cannot be solved using the ordinary integral methods found in most textbooks?

You can quite easily integrate hyperbolic functions - I chose not to as it was beyond the scope of this post.

(5) Wolfram Alpha rendered the results with log and none involving hyperbolic functions. Could it be that what I did was wrong?

Looks OK to me. But we just need a numerical answer, so no need to worry about the algebraic (indefinite) integral.

And finally...

(6) Why not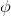 $\phi$ ?

$\phi$ is actually a constant, related to the Golden Ratio. See https://www.wolframalpha.com/input/?i=phi

So Wolfram|Alpha thought you wanted that constant, and didn't realise you were using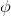 $\phi$ as a variable. Using x, t or theta are usually "safe" variables for computer algebra systems like Wolfram|Alpha.

Hope it helps!
Chris Zuniga says:
25 Jan 2019 at 3:46 pm [Comment permalink]
@Murray

Thanks for your kind words of compliment. In fact I have some experience by using LaTex due I'm constantly asking questions on math in different forums. But so far I found your post the most informative one.

Concerning to the value you referred to 0.22282 I totally overlooked the fact that the OP made a question which "cannot be solved" since apparently assigned an incorrect jump or spacing so that the No. of turns in the spiral to be 7.5 and that cannot be achieved using 0.81, so you made clear later if the distance is 1.4 units then the number of turns would be 7.5. I thought this would apply in my case. But the problem I posed was correctly made. The recent edit you made explained it better and this by extension answers the second question.

However the third question is where I'm still stuck at.

If I do understand correctly in the formula of the spiral what we have is

$r=a+b\theta$

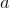with and 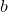being real numbers. If I understood correctly. But if 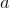is the distance from the center to the point where the spiral begins and this is stated in my case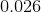

Why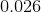 is not "transformed" or "equated" to 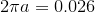$2\pi a = 0.026$ whereas 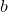in problem is 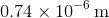$0.74 \times 10^{-6}\,\textrm{m}$ and not 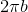$2\pi b$ that's the thing which I'm not getting it.

Could it be that it can be related to this formula?

$s=\theta \times r$ ?

If so what is the justification? Is this used to transform from meters to radians? I'm just as explained confused about the meaning of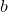 parameter and how is it different from .

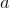Again If that's the case (if it is transformed) I don't understand why one gets its value to stand as it is and the other requires the transformation?. Since they are both real numbers already why one has to change and the other not?. Mind you explaining this to me a bit better?.

You mentioned something about seen it written in both ways. This latter comment I don't understand. What did you meant by written in both ways? Where is the second one?

I am a bit rusty with integration methods but upon an inspection on what I recall I don't find what transformation or change of variable should I use to integrate the function obtained in the end.

When you mentioned that it is okay to you about the logarithmic functions in the resulting integral obtained by Wolfram Alpha did you meant that there was no discrepancy with the supposedly hyperbolic function which should appear in the answer?. How can this happen?.

As mentioned (and i can understand that it was beyond the scope of the question) I'm curious of how to obtain the indefinite integral. Thus I want to try out this excerise on my own using pen and paper.

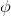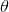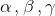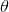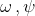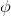Regarding the use of $\phi$ instead of $\theta$ it is a funny story. Back in high school I had an awful experience with trigonometry and as a result I developed an apprehension to $\alpha\,,\beta\,, \gamma$ and more specifically to $\theta$ . Years later when I studied chemistry in university I had this physics course in optics where the notation often used is $\omega\,,\psi\$ and $\phi$ and since that memories bring me more joy (triggered also as I obtained good grades and developed a close friendship with the professor). I prefer to stick to those letters. Maybe it is just a subjective choice but that doesn't really change the meaning of what it was shown. I hope you understand me. However there is nothing wrong with using the "ordinary and usual" letters such as cosines law which is the only exception I do since it is easy to relate the arabic letters with their corresponding greek equivalents.

Out of curiosity one user @Mark suggested to use a much simpler approach

$L=\int^{2\pi N}_{0} r d\theta = \int^{2\pi N}_{0} \left(a+b\theta\right) d\theta$

$L= \left[a\theta + \frac{b \theta^{2}}{2}\right]^{2 \pi N}_{0}$

Now If you don't mind for purposes of brevity and to my mental health I'll rewrite the latter formula into:

$L= \left[a\omega + \frac{b \omega^{2}}{2}\right]^{2 \pi N}_{0}$

(I'm letting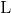 $\textrm{L}$ , because it reminds me the value of inductive resistance from electronics which it happens to be my hobby as well)

Anyways... by plug in the values I obtained earlier this became into:

$N=\frac{31\times 10^{5}\pi}{74}$

$b= \frac{37 \times 10^{-8}}{\pi}$

$L= \left[0.026\omega + \frac{ 37 \times 10 ^{-8} \omega^{2}}{2 \pi}\right]^{2\pi \times \frac{31\times 10^{5}}{74}}_{0}$

To which evaluated using Wolfram Alpha I obtain:

$L= 10923.4\,\textrm{m}$

which amazingly it is very much exactly as what I obtained from your method.

The above method which I posted in my earlier comment was based on the fact that if you consider the area of the spiral to be a flat doughnut then this disk when unwind it results in a parallelogram and therefore the height is considered to be the separation between the arms or the tracks and the length is what it is to be calculated. As indicated this is an approximation and is very similar also to the result. However I'm concerned whether how accurate is this approximation since when you calculate the area by considering that it is a disk there is inevitable some sector which is added in both ends as the spiral doesn't end like a circle does.

I'm sorry If my questions seem redundant but I'd really hope you can help me to clear out these doubts. I'd like to write down this in my notes book to review it frequently.
Murray says:
27 Jan 2019 at 5:22 pm [Comment permalink]
Your questions are certainly not "redundant"! Good on you for pursuing it until you understand it.

"But if is the distance from the center to the point where the spiral begins and this is stated in my case

Why is not "transformed" or "equated" to $2\pi a = 0.026$ whereas in problem is $0.74 \times 10^{-6}\,\textrm{m}$ and not $2\pi b$ that's the thing which I'm not getting it."

Your value of 0.026 is the starting value for your spiral, and if it's divided by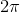 ${2}\pi$ or anything else, then when $\theta={0}$ (that is, when we substitute it), 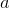won't be your starting value any more.

Could it be that it can be related to this formula?

$s=\theta \times r$ ?

Hopefully this picture helps to explain it.

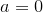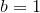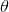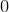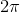I've taken the most fundamental case possible, with and , so the curve is ${r}=\theta$ . As values of $\theta$ go from to ${2}\pi$ , you can see we are at 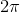${2}\pi$ on the horizontal axis. Similarly if we go around again, we''ll be at 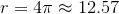${r}={4}\pi\approx{12.57}$ and once more gets us to .

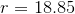That's how polar coordinates work - for whatever value of $\theta$ , we need to move away from the origin by that much.

So since one turn is equivalent to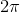 ${2}\pi$ radians, then we need to divide our 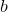in the equation by 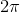${2}\pi$ for it all to work.

If it's still not quite clear, I suggest substituting a bunch of values for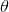 $\theta$ and seeing what values of result.

There are often 2 or 3 ways to find an answer - I'm glad this post surfaced some of them!
Nagesh says:
29 Sep 2019 at 6:27 am [Comment permalink]
Now for a process of oversimplification for the sake of market and the tools on our PC.
Inner dia is known D1, outer dia is known Dn and pitch is known as 'P'. well create a spreadsheet calculator and be done with it.
As many concentric circles as the pitch vs differential of outer dia and inner dia! (Dn-D1)/P etc., It shall be within .2% error, not good enough for space travel but enough for commerce.

I wonder if transformer winding fellows have got it figured?

I was trying to calculate as to how much area of 1.2mm thick leather hide can be packed in a Tube of 120 cm and 20cm dia!
Well, I was wondering if there was a formula for spiral length and here I am!

QED
Dogan says:
13 Jan 2020 at 4:53 am [Comment permalink]
I have the following question:
This sequence formula 1/4 (12 n^2 - 6 n + (-1)^n (4 n - 1) + 1) should indicate the arc length each from zero point on an Archimedean spiral. A point should be created at each end of the arc length. The distances between the spiral tracks are sought. When trying it out experimentally, the points of the arc lengths are roughly arranged at an angle of 120 °. The probably distance is e + 1. how can i calculate it mathematically?
---------------------------------------------------------------
n | \frac{1}{4} (12 n^{2} + 4 (-1)^{n} n - 6 n + (-1)^{n+1} + 1)
1 | 1
2 | 11
3 | 20
4 | 46
5 | 63
6 | 105
7 | 130
8 | 188
9 | 221
10 | 295
11 | 336
12 | 426
13 | 475
14 | 581
15 | 638
Murray says:
14 Jan 2020 at 8:41 pm [Comment permalink]
@Dogan: I'm not sure where that sequence formula comes from, but it doesn't quite give us an Archimedean spiral.

I plotted the points given by the formula (with 120° spacing between each spiral arm) - the dark green segments - and then plotted an actual Archimedean spiral as close as I could to those points - in magenta.

In places it comes close, but mostly it doesn't work well.

The spiral gap is about 130 or so, but it would not be possible to calculate it for the sequence points as they are not evenly spaced.
Simon Geard says:
21 Jun 2020 at 2:19 am [Comment permalink]
The integral for arc length is quite straightforward to obtain using standard techniques (substitution and either integration by parts of double-angle formula for hyperbolic functions). The result is:

${L}=\sigma{{\left[\frac{u}{2}\sqrt{{1}+{u}^{2}}+\frac{1}{2}{\sinh}^{-{1}}{u}\right]}_{{\frac{a}{\sigma}}}^{{\frac{b}{\sigma}}}}$

where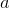 and 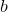are the inner and outer radius of the spiral and $\sigma=\frac{p}{{2}\pi}$ is the increase in radius per radian, 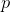is the increase in radius per turn:

${r}={a}+\sigma\theta$

Assuming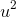 ${u}^{2}$ >> :

${L}\stackrel{\sim}{=}\pi\frac{{b}^{2}-{a}^{2}}{p}+\frac{p}{{4}\pi}{\ln{{\left(\frac{b}{a}\right)}}}$

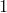For the case: a = 5 , b = 11.075 , p = 0.81 => L = 378.8095

This formula can be adapted for the number of turns by using the fact that

then using the approximation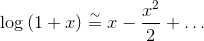 ${\log{{\left({1}+{x}\right)}}}\stackrel{\sim}{=}{x}-\frac{{x}^{2}}{2}+\ldots$ gives a quadratic in :

${L}{\left({n}\right)}\stackrel{\sim}{=}{n}{\left({2}\pi{a}+\frac{{p}^{2}}{{4}\pi{a}}\right)}+{n}^{2}{p}{\left(\pi-\frac{{p}^{2}}{{8}\pi{a}^{2}}\right)}$

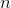provided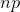 << 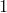since the log series is slow to converge.

For the values above the result is

n L(n) exact
1 33.9708 33.9703
2 73.0304 73.0287
3 117.1789 117.1755
4 166.4162 166.4109
5 220.7424 220.7351
6 280.1574 280.1482
7 344.6612 344.6502
8 414.2539 414.2411
9 488.9354 488.9212
10 568.7058 568.6903
11 653.5650 653.5486
12 743.5130 743.4961
13 838.5499 838.5327
14 938.6756 938.6585
15 1043.8902 1043.8736
16 1154.1936 1154.1779
17 1269.5859 1269.5714
18 1390.0670 1390.0542
19 1515.6369 1515.6263
20 1646.2957 1646.2877

If the spiral is modelled as a series of concentric circles the algebra is much more tedius. The result depends on the value of radius used for each ring. If the value for the i'th ring is

${r}=\alpha{r}_{{i}+{1}}+{\left({1}-\alpha\right)}{r}_{i}$

then

${L}=\pi\frac{{{\left({b}^{2}-{a}^{2}\right)}}}{p}+\pi{\left({b}-{a}\right)}{\left({2}\alpha-{1}\right)}$

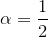with $\alpha=\frac{1}{2}$ corresponding to the average radius. Therefore the length of a spiral calculated using this value will always be an underestimate.
Bill Etienne says:
15 Jul 2020 at 2:22 am [Comment permalink]
I have a problem that is similar to the original:
You have a perfect white sphere one meter in diameter and a remote control that will allow you to roll it in whatever direction you choose with mathematical precision. The sphere is at rest in a wide pool of black ink one centimeter deep. The floor of the pool is a perfectly level plane. What is the minimum distance the sphere must roll to be entirely covered in ink? What does its path look like? The ink does not bleed or run. Once a section of the sphere has touched ink, that section stays inked.

I believe I have the correct answer but I would like to run it by someone else to be sure.