IntMath Newsletter - math symbols, what is math, weekend math study

In this IntMath Newsletter:

1. Math tip (a) – Where did those math symbols come from?
2. Math tip (b) – What is math?
3. Latest IntMath Poll - weekend math study
4. GIS Day on 18 Nov
5. From the Math Blog
6. Final thought – GOALS

==================

1. Math tip (a) – Where did those math symbols come from?

Mary is a subscriber to the IntMath Newsletter. She recently asked:

Dear Sir,
I would like to find the meaning of the bizarre arithmatic symbols, like: * # and many others I do not how to write it on an e-mail.

This is a great question since bad math notation is one of the reasons math appears confusing for many people, and causes unnecessary math anxiety.

Asterisk and other signs for multiplication: The cross sign for multiplication (×) was suggested by the Englishman William Oughtred (1574-1660).

Gottfried Leibniz (1646-1715, the German who developed calculus) rightly objected to the cross because it can easily be confused with the letter "x", which of course is used a lot in math (and which should be typed using Times New Roman font and use italics, like this x, but unfortunately, not enough teachers or book publishers do this, adding to students' confusion).

The "times" sign, or cross (×) is commonly used in England and Commonwealth countries.

Leibniz proposed the dot (. or more commonly the "mid dot", ·) for multiplication and this is used throughout the USA and Europe. (Leibniz also suggested the "cap" symbol (∩) for multiplication. It also has the meaning of "intersection" in set theory.)

Unfortunately, the dot is also used for the decimal point, so if we see "5·3" we should take this to mean "5 times 3", while "5.3" is "5 (decimal) point 3". In Europe it is common to use comma (,) for the decimal point, so 3·2,5 would mean "3 times 2 point 5". This is a little better than if we use dots for both: 3·2.5.

It's all rather silly and confusing.

There is a branch of math where cross product is different from dot product. You can read more here: Dot product of 2 Vectors

The asterisk (*, from the Latin which means "little star") is used in computer programming for multiplication. For example a*b means "a multiplied by b". (It is also used as a "wild card" character, for example *.jpg will find all image files with a "jpg" extension when doing a search.)

Perhaps we should use the asterisk for multiplication in math, too. It is easy to read, easy to type and probably not as prone to confusion as the dot and the × symbols.

The # ("hash", "number sign" or "pound sign"): This symbol also suffers from inconsistent naming and use. According to Wikipedia:

The mainstream use in the U.S. as follows: when it precedes a number, it is read as "number", as in "a #2 pencil" (spoken aloud as: "a number two pencil"); however, when it follows a number it is read as "pounds" referring to the unit of weight, as in "5# of sugar" (spoken aloud as "five pounds of sugar").

This one is not so common in Commonwealth countries, except as a symbol on your telephone.

We should try to simplify and standardize the symbols used in mathematics. Many symbols are used differently in different countries, and often one symbol is used for more than one purpose, and vice-versa.

You may also be interested in Towards more meaningful math notation.

2. Math tip (b) – What is mathematics?

A lot of the time we look at math from a very narrow point of view. Usually we are concerned with the current chapter in our textbook only and have little idea where the math comes from or how it fits into the greater scheme of things.

I've been reading The Math Gene by Keith Devlin. In this book, he argues that humans developed the "math gene" (not a real one, he means the ability to do math) at the same time we developed language. He also believes all people can "do math".

He has a neat way of describing how math developed through history. The answer to "what is mathematics?" is constantly evolving.

Before 500BC - Arthmetic: Math was mostly about numbers and arithmetic. Ancient Egyptian, Babylonian (present-day Iraq) and Chinese mathematics concerned itself with how to combine numbers. It arose from the simple needs of commerce - weighing, counting, measuring, and determining profit.

So mathematics was essentially about numbers.

Between 500 BC and 300 AD - Geometry: The ancient Greeks were very interested in geometry. This was the time of Pythagoras, Euclid and Archimedes, where they tried to solve problems in geometry and ran into some very challenging problems (like what is the value of √2, when you don't believe in irrational numbers? This number appeared when they tried to find the diagonal of a 1×1 square).

Mathematics was the study of numbers and shape during this period.

The 17th century - study of motion and change: Newton (in England) and Leibniz (in Germany) independently developed calculus. This gave a massive impetus for the scientific and engineering achievements of the industrial revolution. It also meant travel by ship became a lot safer and this spurred exploration like never before.

Mathematics became the study of numbers, shape, motion, change and space.

End of the 19th century - tools of math: By the mid-18th century, mathematics took on more of a life of its own, rather than as the supporting cast for physics and the other sciences. There was more interest in studying mathematical phenomena for their own sake.

The answer to "what is math" had become numbers, shape, motion, change, space and the mathematical tools that are used in this study. Whole new branches of math have appeared, like topology, complexity theory, dynamical systems theory, chaos theory and many sub-branches of probability and disaster prediction. Climate change has triggered new interest in modeling and the idea that small inputs can have huge effects.

So a simple answer for the question "what is mathematics?" is that it's the study of patterns. Not just patterns that you see in your carpet or on wallpaper, but patterns in numbers, hierarchies, geometry, language, traffic, algebra, the universe, and so on.

We're all able to spot patterns and to see when a pattern is broken. In fact, that's an important part of being human and for survival. So essentially, we are all mathematicians!

3. Latest IntMath Poll - Weekend math study

A poll on IntMath through October asked readers:

During weekends, how many hours do you spend doing math homework (or study)?

Poll results:

32%  4 or more hours
22%  None
20%  1 hour
17%  2 hours
10%  3 hours

Total votes: 2100
Poll date: Oct 2009

Now, this was an interesting result for me because I know most people do ZERO math on weekends. How do I know?

We can learn a lot from people's behavior on the Web. From their searches in Google, Yahoo or Bing we can see what they really want to know. And from the pages they visit on the Web, we can see where their search took them. How long they stay and how many pages they look at gives us a good indication of what really interests them.

This graph shows total visitor traffic on Interactive Mathematics from Aug to Oct 2009. This is why I asked that question in the poll and why I am surprised at the result.

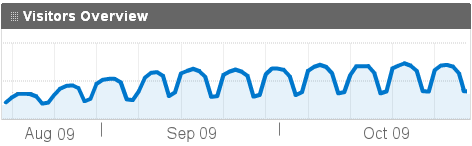
I wrote an analysis of this and some other interesting "math on the Internet" trends in the following special article:

Who studies math on weekends?

4. GIS Day on 18 Nov

There's a lot of interesting real-life math involved in Global Information Systems (GIS), including 3-D Earth Geometry.

Next week is GIS Day, which aims to increase "geographic literacy". From the GIS Day site:

GIS Day is playing a powerful role in creating geographic awareness throughout our world.

GIS Day provides an international forum for users of geographic information systems (GIS) technology to demonstrate real-world applications that are making a difference in our society.

More than 80 countries will participate in holding local events such as corporate open houses, hands-on workshops, community expos, school assemblies, and more.

You can participate at your school or community center. You can get free materials from the site as well. What you learn could help reduce some of the destruction to our planet.

5. From the math blog

a) Who studies math on weekends?
How many students do math homework on the weekends? What caused this spike in search traffic? [This article was mentioned earlier.]

b) Friday Math Movie - Did You Know 4.0
Here's an update to the excellent "Shift Happens" movies.

c) Making math accessible for the blind
The abacus is a useful tool for helping blind people to learn math.

d) MoreNewMath - funny equations about life
Here are some funny and thought-provoking equations explaining life's experiences.

e) 3D Grapher with contour plot
Here's a grapher that will plot your 3D function and will also give you a contour map of it.

6. Final thought - GOALS

The following is based on the work of the motivational guru Brian Tracy, author of the book Eat That Frog! 21 Great Ways to Stop Procrastinating and Get More Done in Less Time.

Only 3% of American adults have a written set of goals. No wonder people have aimless lives.

Goal setting is crucial for success in life - and especially for success in math. Here's a way to get started on your GOALS.

G = Get to It!

There are 2 kinds of people — those who talk about how much they have to do, and those who get on and do it. People wonder why they are unsuccessful at math while sitting around complaining about it.

Set a goal for future math success and then plan how you are going to get there. The Chinese are right when they say, "A journey of a thousand miles begins with a single step".

In short - get to it!

O = Opportunity

Successful people look for opportunities or create their own. Sure, there may be things standing in the way of your success, but there are huge opportunities as well. For a start, the Web provides really good free resources in math, and plenty of excellent paid ones. Perhaps you could look for people in your area that have math expertise. Maybe some retired math teacher could give you help in exchange for doing some chores.

A = Ability

Many people feel that just a select few can do math and forget it for the rest. But you can count, can't you? You can add and multiply? You can recognize patterns? Well, of course you have the ability to do math. The difference between an Olympic runner and a weekend jogger is not that huge - the difference is in the goals they have set themselves.

Perhaps this one should have been called A = Attitude instead.

L = Leadership

This one refers to leading yourself to where you want to go. No point blaming your teacher (or parents, boss, or coach). What you do with your own goals is a strong indicator of your leadership abilities.

S = Stay With It!

Successful people are the ones who stick it out and grow when things go wrong. You'll make mistakes in math for sure (I've made hundreds of them). They'll be frustrating, but by going back and fixing them, you learn more and become even stronger at math.

So spend some time to write down your goals and your plan for achieving those goals one step at a time. You'll be way ahead of the guy who just drifts along.

Good luck with your goal setting!

Until next time.

See the 14 Comments below.