Skip to main content
Interactive Mathematics
Search IntMath
Close

IntMath Newsletter: Interactive 3D graph, Polymath

By Murray Bourne, 19 Apr 2016

19 Apr 2016

In this Newsletter:

1. New 3D interactive graph
2. Over 70 interactives
3. Roller door problem and Polymath software
4. Math in the news
5. Math puzzles
6. Math movie: Math symbols in ancient rock art?
7. Final thought: Mathematical habits of mind

1. New 3D interactive graph

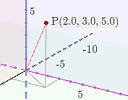
3D interactive graph applet - background

I recently added a simple 3D interactive graph which allows you to explore the x-, y- and z-axis system. Here's some background (which includes a discussion about the "correct" orientation for the axes in a 3D graph):

New 3D interactive graph

2. Now over 70 interactives!

Math applets on IntMath

There are now over 70 interactive math applets (most of them mobile-friendly) on IntMath.

I recently updated the full list, which you can find here:

Math Interactive Applets

3. Roller door problem and Polymath software

Polymath roller door problem

Here's a numerical solution to an earlier problem we discussed involving the height of a roller door given the number of turns the door makes.

Roller door problem and Polymath software

4. Math in the News

(a) Andrew Wiles receives Abel Award for solving Fermat's Last Theorem

Andrew Wiles wins Abel Prize

Sir Andrew Wiles solved Fermat's Last Theorem in 1994. He proved there are no integer solutions for n ≥ 3 in the equation xn + yn = zn.

Wiles was recently awarded the Abel Prize "for his stunning proof of Fermat’s Last Theorem by way of the modularity conjecture for semistable elliptic curves, opening a new era in number theory".

His prize money is equivalent to $850,000 (USD).

Last year's joint winners for the Abel Prize were John F. Nash Jr. (who was the subject of the movie A Beautiful Mind) & Louis Nirenberg.

(b) Math Awareness Month: Making Predictions

Making Predictions - Math Awareness Month

April is Math Awareness Month, and the theme this year is "Making Predictions".

It's a good theme, and one that we should encourage more in mathematics courses. Making predictions requires mathematical modelling which is an incredibly important concept, but is rarely taught in schools.

The articles on the past, present and future of prediction and the central position of mathematics and statistics are quite interesting.

5. Math puzzles

The puzzle in the last IntMath Newsletter asked about a journey by two girls involving a bicycle and/or walking.

Correct answers with sufficient explanation were given by: Chris and Hamid. There was certainly an interesting assortment of solutions for this one!

New math puzzle

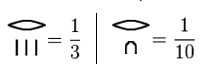
Egyptian fractions

The Egyptians used unit fractions, where the numerator was always 1.

They would need to write their ratios as the sum (not difference) of unique unit fractions, like this:

\frac{3}{5}=\frac{1}{2}+\frac{1}{10}

Using this system, how would they have written \frac{11}{13}?

You can leave your responses here.

6. Math movie: Geometric shapes in ancient rock art paintings

rock art

Ancient rock art may give clues to the origin of written symbols, including early math notation.

Geometric shapes in ancient rock art paintings

7. Final thought: Mathematical habits of mind

In the article Mathematical Habits of Mind, Cindy Bryant discusses the importance of developing sound strategies for solving math problems. Expert problem solvers don't panic when they come across an unfamiliar problem. They will use one or more of the following strategies (based on Polya''s four-step problem solving process) until something "clicks":

Draw a picture
Find a pattern
Make a list
Make a table
Solve a simpler problem
Guess and check
Act out the problem
Work backwards
Write an equation

Until next time, enjoy whatever you learn.

Image credits for this Newsletter:

See the 15 Comments below.

Related posts:

  1. IntMath Newsletter: Derivative graphs, roller door problem, online math in remote India In this Newsletter: 1. Derivative graphs interactive 2. Roller door problem 3. Online math education...
  2. Roller Door Problem and Polymath Software Here's a numerical solution to an earlier problem we discussed involving a roller door....
  3. IntMath Newsletter: Fourier graph, resources In this Newsletter: 0. Top 100 Math Blog 1. Fourier Series interactive graph 2. Why...
  4. IntMath Newsletter: z-Table interactive graph, CK-12 resource, range hood In this Newsletter: 1. New z-Table interactive graph 2. Resource: CK-12.org 3. Math puzzles 4....
  5. IntMath Newsletter: Interactive ellipses, Wolfram Education Portal In this Newsletter: 1. Interactive ellipse graphs 2. Wolfram's Education Portal 3. Puzzle 4. IntMath...

Posted in Intmath Newsletters category - 19 Apr 2016 [Permalink]

15 Comments on “IntMath Newsletter: Interactive 3D graph, Polymath”

  1. cccccttttt says:
    19 Apr 2016 at 11:15 am [Comment permalink]

    Subtract 1/2 from 11/13.
    From that subtract 1/3.
    This last difference is 1/78.

    (1/2)+(1/3)+(1/78)= 11/13

    No wonder only priests and builders used fractions.

    -----------------

    Well done 3D applet.

    Far better illusion than my hand drawings.

    You may eventually offer an option
    to viewers with VR googles.

    ct

  2. Mike Thayer says:
    19 Apr 2016 at 6:18 pm [Comment permalink]

    Good problem!

    11/13 = 1/2 + 1/3 + 1/78

  3. Nour Hlwani says:
    20 Apr 2016 at 1:27 am [Comment permalink]

    We know that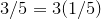 3/5 = 3(1/5)
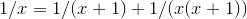
    And that 1/x = 1/(x+1) + 1/(x(x+1))

    So we conclude that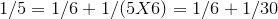 1/5 = 1/6 + 1/(5 X 6) = 1/6 + 1/30

    And finally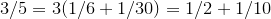 3/5 = 3(1/6 + 1/30) = 1/2 + 1/10

    Using the same technique for 11/13
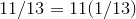
    11/13 = 11(1/13)
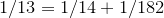
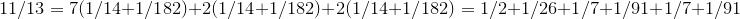
    And 1/13 = 1/14 + 1/182
    Then 11/13=7(1/14+1/182)+2(1/14+1/182)+2(1/14+1/182) =1/2+1/26+1/7+1/91+1/7+1/91

    We still need to clear the duplicates
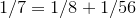
    1/7 = 1/8+1/56
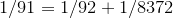
    1/91=1/92+1/8372

    And the answer would be
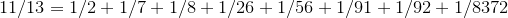
    11/13 = 1/2 + 1/7 + 1/8 + 1/26 + 1/56 + 1/91 + 1/92 + 1/8372

  4. Don Miller says:
    20 Apr 2016 at 2:42 am [Comment permalink]

    1/2 + 1/3 = .833333 close to 11/13

    let x= 11/13 - .833333, and y =1/x

    By searching, y=78. So 1/2 +1/3 + 1/78 = 11/13

  5. Lidia Carro says:
    20 Apr 2016 at 10:08 am [Comment permalink]

    1/2 + 1/3 + 1/78 = 11/13

  6. Rika says:
    20 Apr 2016 at 3:07 pm [Comment permalink]

    It is more than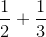 \frac{1}{2}+\frac{1}{3},this minus and get the answer:
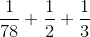
    {\frac{{1}}{{78}}}+{\frac{{1}}{{2}}}+{\frac{{1}}{{3}}}

  7. Rika says:
    20 Apr 2016 at 3:09 pm [Comment permalink]

    Hi Murray
    Hope you are well. I am still so thankful to you for your help with maths on internet 🙂
    Regards
    Rika

  8. Murray says:
    20 Apr 2016 at 7:08 pm [Comment permalink]

    I'm well, Rika, and you're welcome!

  9. Ben Murray says:
    21 Apr 2016 at 7:58 pm [Comment permalink]

    The unit fractions, in decreasing order of size, are 1/2, 1/3, 1/4, 1/5 and so on.

    11/13, the fraction that we need to convert to unit fractions, Egyption-style, is nearly one. Definitely bigger than 1/2, so let's subtract 1/2 and see what we're left with:

    11/13 - 1/2 = 22/26 - 13/26 = 9/26.

    So we know that 11/13 = 1/2 + 9/26. We're making progress, but there's still more work to do.

    9/26 is just a tiny bit bigger than 1/3, so let's take that away and see what remains:

    9/26 - 1/3 = 27/78 - 26/78 = 1/78. Which, happily enough, is also a unit fraction.

    Putting it all together, we can see that:

    11/13 = 1/2 + 1/3 + 1/78. Numbers that the Eqyptians can relate to.

  10. karam says:
    21 Apr 2016 at 9:01 pm [Comment permalink]

    So thank you for this wonderful effort
    11/13 = 1/2 + 1/4 + 1/13 + 1 /52

  11. Kjell O. Foss says:
    24 Apr 2016 at 2:40 am [Comment permalink]

    11/13 = 1/2 + 1/4 + 1/13 + 1/52

  12. george says:
    27 Apr 2016 at 11:00 am [Comment permalink]

    1/2 + 1/3 + 1/26 = 11/13

  13. Thomas A Buckley says:
    5 May 2016 at 5:35 am [Comment permalink]

    11/13
    = 22/26
    = 13/26 + 9/26
    = 1/2 + 18/52
    = 1/2 + 13/52 +5/52
    = 1/2 +1/4 + 4/52 +1/52
    = 1/2 + 1/4 + 1/13 + 1/52
    =
    ............
    I I + IIII + n + III + n+n+n+n+n + II

    Graphic limited ! Bah

  14. Thomas A Buckley says:
    5 May 2016 at 5:52 am [Comment permalink]

    My graphic looked OK in preview comment,
    but lost numerator symbols when submitted.
    So for clarity I've separated my 4 fractions, omitting the numerator symbol.

    II, IIII, n+III, n+n+n+n+n+II
    Furthermore the +'s may be omitted, if the numerator symbols are assumed to be added.
    Yielding,
    II, IIII, nIII, nnnnnII

  15. Jess Grindlay says:
    24 May 2016 at 11:30 pm [Comment permalink]

    I split it into 3, and I got:
    1/3 + 1/2 + 1/78

    I times it through by 3, so I could split it into 1 third and then got a half from that and realised the remainder was only one.

Leave a comment




Comment Preview

HTML: You can use simple tags like <b>, <a href="...">, etc.

To enter math, you can can either:

  1. Use simple calculator-like input in the following format (surround your math in backticks, or qq on tablet or phone):
    `a^2 = sqrt(b^2 + c^2)`
    (See more on ASCIIMath syntax); or
  2. Use simple LaTeX in the following format. Surround your math with \( and \).
    \( \int g dx = \sqrt{\frac{a}{b}} \)
    (This is standard simple LaTeX.)

NOTE: You can mix both types of math entry in your comment.

top

Tips, tricks, lessons, and tutoring to help reduce test anxiety and move to the top of the class.