IntMath Newsletter: Forum, pistons, Shodor
By Murray Bourne, 26 Jan 2016
26 Jan 2016
In this Newsletter:
1. New: IntMath Forum
2. Piston curve
3. Resource: Shodor Interactivate
4. Math puzzles
5. Math movies: Misleading statistics
6. Final thought: Resolutions, 1% at a time
1. New: IntMath Forum
A lot of people have asked for a forum over the years. Finally, it's here!
I come across too many low quality forums and I'm hoping to avoid that. I also get a lot of mails containing math homework questions where the writer just wants me to provide the answer. This doesn't help him learn much - it just helps his marks. So there are 5 simple principles for the forum:
Principles of the IntMath Forum
You can enter math in the forum using simple plain text (or LaTeX if you prefer). You can also add graphs and images to your post. Details on how to do so can be found here:
How to enter math, graphs and images
Wanted: Volunteer Tutors
The forum needs tutors that embrace the 5 Principles mentioned above. That is, tutors should firstly be confident in answering the question, but help readers figure out problems by themselves, not just do it for them. The best help is given by prompting, reminding, and asking good questions - not simply providing the answer.
To get an idea what I mean, see these exchanges where I'm pointing the user to relevant resources, and asking questions. Eventually, they work it out:
- Graphing a Fish Population Word Problem
- Lowest common denominator
- Prove the trig identity cosx/(secx+tanx)= 1-sinx
Let me know (by replying to this mail, or in the comments) if you are interested to be a tutor.
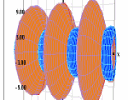
2. Does a piston follow a sine curve shape?
 |
In the Trigonometric Graphs section, I used a piston example for one of the sine curves. A reader pointed out that a piston does not exactly follow a sine curve at all. Here's a short article with an interactive animation that explores this issue: |
3. Resource: Shodor's Interactivate
 |
I've been a fan of Shodor's Interactivate for many years. Indeed, it helped to inspire IntMath. Their activities used to be java-based, and so didn't work on mobile devices. But now, their interactive activities are mobile friendly. See over 300 activities, covering algebra, functions, ciphers, calculus, and probability here: |
4. Math puzzles
The puzzle in the last IntMath Newsletter asked about the ratio of the areas of Reuleaux Triangles.
Correct answer with explanation was given by Stephen, who was also was one of the few to express the final answer as a ratio, as required.
New math puzzle
The length of a rectangle increases by 10% and the width decreases by 10%. Which of the following is true?
- The area does not change.
- The area becomes smaller or larger depending on the original lengths of the sides.
- The area will always become smaller.
- The area will always become larger.
You can leave your responses here.
5. Math movie: Misleading statistics
 |
This video outlines Simpson's Paradox, which all budding statisticians need to be aware of. This is part of a TED-Ed lesson. |
Related article: Misleading charts
Around the same time I watched How statistics can be misleading, I came across the following article on Quartz.
I feel it's a vital mathematical skill to be able to properly analyze data, especially when it's in visual form.
I don't agree with all the writer's suggestions, but overall it's quite a good analysis:
The most misleading charts of 2015, fixed
6. Final thought: Resolutions, 1% at a time
You probably made new year resolutions and now that life has taken over, maybe they've been shelved already.
A possibly more effective and realistic way of challenging yourself to improve is to aim for 1% improvement per day (or some other reasonably short time frame). If you want to exercise more, try just walking (or running, or any other exercise) 1% more than the day before. This will mean you'll double your walking distance in just 70 days:
(1.01)70 = 2.007
You could apply a similar small and realistic improvement goal for your studies: say 5% improvement for each weekly test.
Either way, all the best achieving your goals in 2016!
Until next time, enjoy whatever you learn.
See the 17 Comments below.
26 Jan 2016 at 7:51 pm [Comment permalink]
Original Area = LW
New Area = (1.1 L)(.9 W) = .99 LW
The combination of the two changes always results in a decrease in the original area.
26 Jan 2016 at 8:22 pm [Comment permalink]
Solution of the puzzle:
The area will always become smaller.
Explanation:
Let the length of the rectangle= x
and the width of the rectangle= y
Clearly, x>0, y>0
So the area of the rectangle= x*y
Now,
new length= x+(x/10)= (11*x)/10
new width= y-(y/10)= (9*y)/10
So the new area= (11x/10)*(9y/10)= 0.99*xy, which is always less than xy.
27 Jan 2016 at 1:18 am [Comment permalink]
Rectangular Areas
Let the area of the original rectangle be
If we increase the length by 10%, we have a new length
given by
Similarly, the new width will be
The new rectangle then will have an area of
So the new area will always be 99% of the old area - a 1% decrease
27 Jan 2016 at 1:32 am [Comment permalink]
Area will always become larger. (As length > width of a rectangle and area = length * width)
27 Jan 2016 at 1:48 am [Comment permalink]
Let L = original length and W = original width. Original area = L*W.
Length increases by 10%, so new length = 1.1*L
Width decreases by 10%, so new width = 0.9*W
New area = (1.1*L)(0.9*W) = 0.99*LW
Therefore, the new area is always 99% of the old area, so the new area is always smaller than the old area.
27 Jan 2016 at 3:55 am [Comment permalink]
Always smaller
27 Jan 2016 at 9:11 am [Comment permalink]
The area wll always become smaller. Call w the width and w + k the length (k > 0). Then the area is A = w (w + k). If the length is increased by 10% and the width decreased by 10%, the resulting area is 0.9 w times 1.1 (w + k) = 0.99 w (w + k) = 0.99 A, which is less than A.
27 Jan 2016 at 3:30 pm [Comment permalink]
The areas will become :
Smaller or larger depending on the original values.
27 Jan 2016 at 9:26 pm [Comment permalink]
Consider a rectangle with the length a and width b.
, so 
Area before change
The length a increases by 10% so
The width b decreases by 10% so
Area after change
So the area will always become smaller.
28 Jan 2016 at 2:31 am [Comment permalink]
Let the length be a and width b. Then the area E=a*b. If a is increased 10% and b decreased 10%, then a'=1.1*a and b'=0.9*b. The new area E'=a'*b'=1.1*a*0.9*b=0.99*a*b, so E'=0.99*E and the area will always become smaller, the 99% of the initial area.
28 Jan 2016 at 5:40 am [Comment permalink]
cute
The area will always become smaller.
28 Jan 2016 at 5:26 pm [Comment permalink]
Hello,
The math puzzle...
The area will become smaller.
Take a rectangle 200 cms long with width 100 cms.
200 X +10% = 220 cms long, 100 X -10% = 90 cms width.
Multiply the original size out, subtract from the new size; the new rectangle has a smaller area.
Kind regards,
Roger
28 Jan 2016 at 8:45 pm [Comment permalink]
The area will always become smaller and exactly by 1% .
Area = L*B ----> 1.1L*0.9B = 0.99L*B
29 Jan 2016 at 6:26 am [Comment permalink]
Area of A rectangle =
Where L --> length and l --> width
L increased by 10% ->
l decreased by 10% ->
The new area = 1.1×0.9×L×l = 0.99×(old area)
Since 0.99<1 Area is slightly decreasing
The right answer is (the area will alway become smaller.
1 Feb 2016 at 3:53 pm [Comment permalink]
The new area will always be smaller
i.e. new area= 0.99 * original area
3 Feb 2016 at 6:27 am [Comment permalink]
The area will always get smaller, no matter which number is called length and which is width. New area = (L + 0.1L)(W - 0.1W) = LW + 0.1LW - 0.1LW - 0.01LW = LW - 0.01LW. So the new area is always one percent smaller than the original area LW. (This seems too easy...what did I miss here?)
16 Feb 2016 at 2:43 pm [Comment permalink]
The area of the triangle will automatically reduce.the reduction will b an approximation of 1% as compared to the previous one.