The Math Behind the Beauty
By M. Bourne
Jessica Simpson
What has mathematics got to do with beauty? Actually, a lot. Physical attraction depends on ratio.
Our attraction to another person's body increases if that body is symmetrical and in proportion. Likewise, if a face is in proportion, we are more likely to notice it and find it beautiful.
Scientists believe that we perceive proportional bodies to be more healthy.
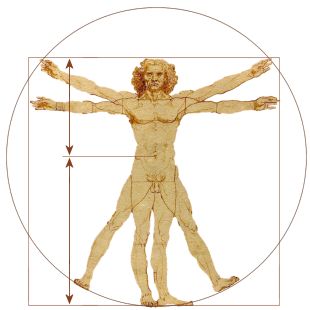
Leonardo da Vinci's "Vitruvian Man",
showing the golden ratio in body dimensions
Leonardo da Vinci's drawings of the human body emphasised its proportion. The ratio of the following distances is the Golden Ratio:
(foot to navel) : (navel to head)
Similarly, buildings are more attractive if the proportions used follow the Golden Ratio.
Golden Ratio
The Golden Ratio (or "Golden Section") is based on Fibonacci Numbers, where every number in the sequence (after the second) is the sum of the previous 2 numbers:
1, 1, 2, 3, 5, 8, 13, 21, ...
We will see (below) how the Fibonnaci Numbers lead to the Golden Ratio:
Φ = 1.618 033 ...
Physical Beauty
Why do many people feel that Jessica Simpson is beautiful?
This mask of the human face is based on the Golden Ratio. The proportions of the length of the nose, the position of the eyes and the length of the chin, all conform to some aspect of the Golden Ratio.
When placed over the photo of Jessica Simpson, we see there is a good fit (that is, the proportions of her face fit the geometrically "nice" proportions of the mask, based on the Golden Ratio).
Her beauty is mathematical!
You can play with this concept in the following interactive.
Flash Interactive
In this Flash activity, you can check out the "mathematical beauty" (or not) of some famous people.
Choose a mask, depending on whether the person is smiling or not, and line up the mask with the photo, using the sliders.
You can change the colour of the mask for better viewing.
This interactive is based on the work of Dr. Stephen Marquardt (external site).
Further Information
In Elle Magazine, the article "Tech Support" describes the work of Tommer Leyvand, a computer scientist who developed a method of "digital face beautification" using software. You can see some examples of his work at Digital Face Beautification and there's a short PDF explaining the math behind the technique.
Disclaimer: Of course, inner beauty is more important than external beauty...!
Back to the Mathematics...
Let's look at the ratio of each number in the Fibonacci sequence to the one before it:
`1/1 = 1`
`2/1 = 2`
`3/2 = 1.5`
`5/3 = 1.666...`
`8/5 = 1.6`
`13/8 = 1.625`
`21/13 = 1.61538...`
`34/21 = 1.61905...`
`55/34 = 1.61764...`
`89/55 = 1.61861...`
If we keep going, we produce an interesting number which mathematicians call "phi" (Golden Ratio or Golden Section):
`Phi = 1.618 033 988 7...`
This ratio was used by architects and artists throughout history to produce objects of great beauty (like Michelangelo's "David" and the Greek temples.)
The Parthenon in Greece. The ratio of the distances indicated is the Golden Ratio.
Phi (Φ) is like pi (π) in the sense that it is an irrational number. There is no equivalent fraction for Φ and its decimal keeps going and never stops.
The Golden Ratio also occurs in nature, in the patterns we see in sunflowers, pine cones and so on. This is largely because one of the best ways to efficiently pack things tightly together is using the Fibonacci sequence.
Related Sections in Interactive Mathematics
You may also be interested in:
- Fractals - intriguing designs made using mathematics
- Nautilus shells - whose shape involves exponentials and trigonometry
- Lissajous figures - more math-based patterns
- Digital audio and light - CDs and light spectrums
- The Math of the Moors - about the people who greatly influenced art and architecture in Europe
- Pi
Didn't find what you are looking for on this page? Try search:
Online Algebra Solver
This algebra solver can solve a wide range of math problems. (Please be patient while it loads.)
Go to: Online algebra solver
Ready for a break?
Play a math game.
(Well, not really a math game, but each game was made using math...)
The IntMath Newsletter
Sign up for the free IntMath Newsletter. Get math study tips, information, news and updates each fortnight. Join thousands of satisfied students, teachers and parents!
Share IntMath!
Short URL for this Page
Save typing! You can use this URL to reach this page:
intmath.com/beauty
Algebra Lessons on DVD
Easy to understand algebra lessons on DVD. See samples before you commit.
More info: Algebra videos
