10. Bayes' Theorem
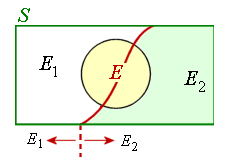
Let E1 and E2 be two mutually exclusive events forming a partition of the sample space S and let E be any event of the sample space such that P(E) ≠ 0.
On this page...
Example 1
The sample space S is described as "the integers `1` to `15`" and is partitioned into:
E1 = "the integers `1` to `8`" and
E2 = "the integers `9` to `15`".
If E is the event "even number" then we have the following:
[Recall from Conditional Probability that the notation P(E1 | E) means "the probability of the event E1 given that E has already occurred".]
Statement of Bayes' Theorem
The probabilities for the situation described above is given by Bayes' Theorem, which can be calculated in two ways:
Method 1
`P(E_1 | E)=(P(E_1nnnE))/(P(E_1nnnE)+P(E_2nnnE))`
`=(P(E_1)xxP(E|E_1))/(P(E_1)xxP(E|E_1)+P(E_2)xxP(E|E_2)`
So for our example above, checking both items of this equation:
`P(E_1|E)=(P(E_1nnnE))/(P(E_1nnnE)+P(E_2nnnE))`
`=(4/15)/(4/15+3/15)`
`=4/7`
Method 2
We get the same result using the second form:
`P(E_1|E)=(P(E_1)xxP(E|E_1))/(P(E_1)xxP(E|E_1)+P(E_2)xxP(E|E_2))`
`=(8/15xx4/8)/(8/15xx4/8+7/15xx3/7)`
`=(4/15)/(7/15)`
`=4/7`
Extending Bayes' Theorem for Mutually Exclusive Events
Bayes' Theorem can be extended as follows:
If E1, E2, ... , Ek are mutually exclusive events forming partitions of the sample space S and if E is any event of S such that P(E) ≠ 0, then
`P(E_i|E)=(P(E_i nnnE))/(P(E_1nnnE)+P(E_2nnnE)+...+P(E_knnnE))`
Example 2
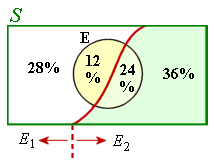
Of all the smokers in a particular district, `40%` prefer brand `A` and `60%` prefer brand `B`. Of those smokers who prefer brand `A`, `30%` are females, and of those who prefer brand `B`, `40%` are female. What is the probability that a randomly selected smoker prefers brand `A`, given that the person selected is a female?
Answer
S = "smokers in the district",
E1 = "prefer brand A",
E2 = "prefer brand B" and
E is the event "female".
`P(E_1|E)=(P(E_1nnnE))/(P(E_1nnnE)+P(E_2nnnE))`
`=(12/100)/(12/100+24/100)`
`=1/3`
Or equivalently:
`P(E_1|E) = (P(E_1)xxP(E|E_1))/(P(E_1)xxP(E|E_1)+P(E_2)xxP(E|E_2))`
`=(0.4xx0.3)/(0.4xx0.3+0.6xx0.4)`
`=1/3`
Example 3
There are 3 urns `A`, `B` and `C` each containing a total of `10` marbles of which `2`, `4` and `8` respectively are red. A pack of cards is cut and a marble is taken from one of the urns depending on the suit shown - a black suit indicating urn `A`, a diamond urn `B`, and a heart urn `C`. What is the probability a red marble is drawn?
If somebody secretly cut the cards and drew out a marble and then announced to us a red marble had in fact been drawn, could we compute the probability of the cut being, say, a heart (or more generally, can we compute the probability of a specified prior event given that the subsequent event did occur)?
Answer
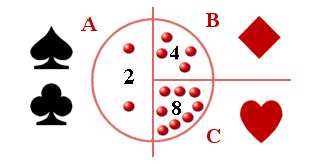
`P(R)=P(A)xxP(R|A)+` `P(B)xxP(R|B)+` `P(C)xxP(R|C)`
`=26/52xx2/10+` `13/52xx4/10+` `13/52xx8/10`
`=2/5`
`P(H|R)` `=(P(H)xxP(R|H))/(P(R))` `=(13/52xx8/10)/(4/10)` `=1/2`
`P(D|R)` `=(P(D)xxP(R|D))/(P(R))` `=(13/52xx4/10)/(4/10)` `=1/4`
`P(Bl|R)` `=(P(Bl)xxP(R|Bl))/(P(R))` `=(26/52xx2/10)/(4/10)` `=1/4`
Conclusion: The probabilities add to 1, so we can compute the probability of a specified event given the subsequent event did occur.
Further explanation of Bayes' Theorem
Here's 2 video explanations of Bayes' Theorem: